5 Compounded Continuously Effective Rate of Interest

Effective Interest Rate Definition

Effective interest Rate, also known as annual equivalent rate, is the rate of interest that is actually paid or earned by the person on the financial instrument which is calculated by considering the effect of the compounding over the period of the time.

Table of contents

• Effective Interest Rate Definition
  • Effective Interest Rate Formula
  • Interpretations
  • Example
    • Example#1
    • Example#2
    • Example#3
  • Effective Interest Rate in Excel
  • Effective Interest Rate Video
  • Recommended Articles

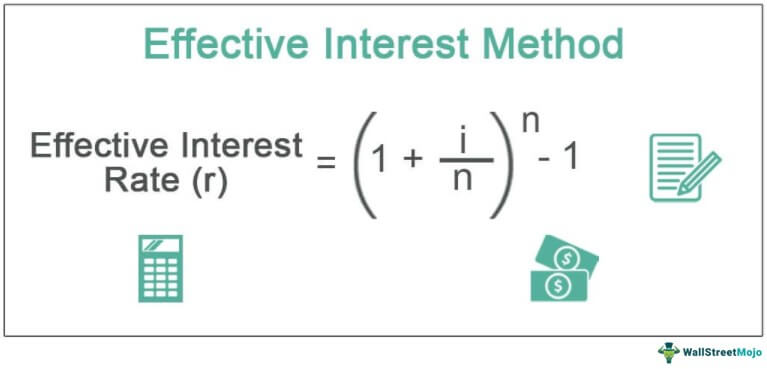
Effective Interest Rate Formula

Effective Interest Rate Formula = (1 + i/n) ⁿ – 1

You are free to use this image on your website, templates, etc, Please provide us with an attribution link Article Link to be Hyperlinked
For eg:
Source: Effective Interest Rate (wallstreetmojo.com)

Here, i = the annual interest rate that has been mentioned in the instrument.

n = It represents the number of compounding periods per year.

Interpretations

Compounding changes the interest rate. That's why the interest rate written on the instrument isn't an effective interest rate (annual equivalent rate) for the investor. For example, if an 11% interest rate is written on the instrument and the interest rate gets compounded four times a year, then the annual equivalent rate can't be 11%.

What would it be then?

It would be – (1 + i/n) ⁿ – 1 = (1 + 0.11/4) ⁴ – 1 = 1.1123 – 1 = 0.1123 = 11.23%.

That means 11.23% would be the effective interest rate for the investor.

Even if the change is meager, it's not the same as the annual interest rate mentioned in the instrument.

Example

Example#1

Ting bought a particular instrument. The interest rate mentioned in the instrument is 16%. He has invested around $100,000. The instrument compounds annually. What would be the effective interest rate (AER) for this particular instrument? How much would he get every year as an interest?

The effective interest rate and the annual rate aren't always the same because the interest gets compounded a number of times every year. Sometimes, the interest rate gets compounded semi-annually, quarterly, or monthly. And that's how the annual equivalent rate differs from the annual interest rate.

This example shows you that.

Let's calculate.

Since the interest rate gets compounded yearly, here's would be the effective interest rate formula –

(1 + i/n) ⁿ – 1 = (1 + 0.16/1) ¹ – 1 = 1.16 – 1 = 0.16 = 16%.

That means, in this particular example, there would be no difference between the annual interest rate and an annual equivalent rate (AER).

Every year Ting would get the interest of = ($100,000 * 16%) = $16,000 on the instrument.

Example#2

Tong bought a particular instrument. The interest rate mentioned in the instrument is 16%. He has invested around $100,000. The instrument compounds six times a year. What would be the annual equivalent rate (AER) for this particular instrument? How much would he get every year as an interest?

This is just an extension of the previous example.

But there's a huge difference.

In the previous example, the instrument got compounded once a year, which made the annual interest rate similar to the annual equivalent rate.

However, in this case, the scenario is completely different.

Here we have the interest rate that gets compounded six times a year.

So, here's the formula of the annual interest rate –

(1 + i/n) ⁿ – 1 = (1 + 0.16/6) ⁶ – 1 = 1.171 – 1 = 0.171 = 17.1%.

You can now see that if the interest rate gets compounded six times a year, the annual equivalent rate becomes quite different.

Now, as we have an effective interest rate, we can calculate the interest Tong will get at the end of the year.

Tong will get = ($100,000 * 17.1%) = $17,100.

If we compare the interest, Ting gets in the previous example with the Tong gets as the interest rates compound. Differently, we will see that there are around $1100 of difference in interest.

Example#3

Ping has invested in an instrument. She has invested $10,000. The interest rate mentioned in the instrument is 18%. The interest gets compounded monthly. Find out how, in the first year, Ping will receive interest every month.

This is a much detailed example of the annual equivalent rate.

In this example, we will show how the calculation actually happens without using the Effective Interest Rate formula.

Let's have a look.

Since the interest rate gets compounded monthly, the actual break-up of the mentioned interest rate per month is = (18/12) = 1.5%.

• In the first month, Ping will receive an interest of = (10,000 * 1.5%) = $150.
• In the second month, Ping will receive an interest of = {(10,000 + 150) * 1.5%} = (10,150 * 1.5%) = $152.25.
• In the third month, Ping will receive an interest of = {(10,000 + 150 + 152.25) * 1.5%} = (10,302.25 * 1.5%) = $154.53.
• In the fourth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53) * 1.5%} = (10,456.78 * 1.5%) = $156.85.
• In the fifth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85) * 1.5%} = (10,613.63 * 1.5%) = $159.20.
• In the sixth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20) * 1.5%} = (10,772.83 * 1.5%) = $161.59.
• In the seventh month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59) * 1.5%} = (10,934.42 * 1.5%) = $164.02.
• In the eighth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02) * 1.5%} = (11098.44 * 1.5%) = $166.48.
• In the ninth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02 + 166.48) * 1.5%} = (11264.92 * 1.5%) = $168.97.
• In the tenth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02 + 166.48 + 168.97) * 1.5%} = (11433.89 * 1.5%) = $171.51.
• In the eleventh month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02 + 166.48 + 168.97 + 171.51) * 1.5%} = (11605.40 * 1.5%) = $174.09.
• In the twelfth month, Ping will receive an interest of = {(10,000 + 150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02 + 166.48 + 168.97 + 171.51 + 174.09) * 1.5%} = (11779.49 * 1.5%) = $176.69.

The total interest Ping got for the year is –

• (150 + 152.25 + 154.53 + 156.85 + 159.20 + 161.59 + 164.02 + 166.48 + 168.97 + 171.51 + 174.09 + 176.69) = $1956.18.
• Annual Equivalent Rate  formula = (1 + i/n) ⁿ – 1 = (1 + 0.18/12) ¹² – 1 = 1.195618 – 1 = 0.195618 = 19.5618%.

So, the interest Ping would receive = ($10,000 ^ 19.5618%) = $1956.18.

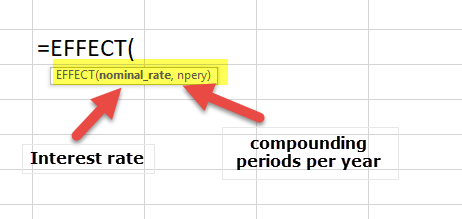
Effective Interest Rate in Excel

For finding the Effective Interest Rate or Annual Equivalent Rate in excel, we use the excel Function EFFECT.

You are free to use this image on your website, templates, etc, Please provide us with an attribution link Article Link to be Hyperlinked
For eg:
Source: Effective Interest Rate (wallstreetmojo.com)

• nominal_rate is the interest rate
• nper is the number of compounding periods per year

Let us see the example below

You are free to use this image on your website, templates, etc, Please provide us with an attribution link Article Link to be Hyperlinked
For eg:
Source: Effective Interest Rate (wallstreetmojo.com)

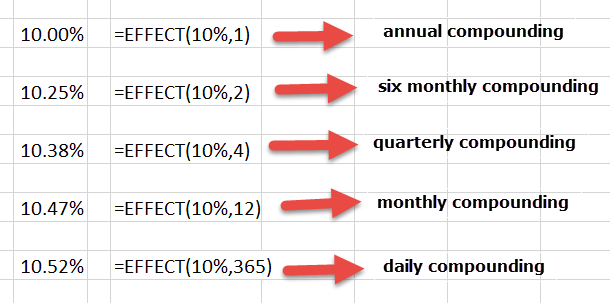
• If you have a nominal interest rate of 10% compounded annually, then the Annual Equivalent Rate is the same as 10%.
• If you have a nominal interest rate of 10% compounded six-monthly, then the Annual Equivalent rate is the same as 10.25%.
• If you have a nominal interest rate of 10% compounded quarterly The compounding quarterly formula depicts the total interest an investor can earn on investment or financial product if the interest is payable quarterly and reinvested in the scheme. It considers the principal amount, quarterly compounded rate of interest and the number of periods for computation. read more , then the Annual Equivalent rate is the same as 10.38%.
• If you have a nominal interest rate of 10% compounded monthly, then the Annual Equivalent rate is the same as 10.47%.
• If you have a nominal interest rate of 10% compounded daily, then the effective interest rate is the same as 10.52%.

Effective Interest Rate Video

Recommended Articles

This article was the guide to Effective Interest Rate and its definition. Here we discuss the formula of Effective Interest Rate along with step by step calculations. For further learnings, you may refer to the following articles

• Negative Interest Rate Example
• Calculate Participation Rate
• Differences – Discount Rate vs. Interest Rate
• Nominal Interest Rate Formula
• Cointegration

cadeloortambel.blogspot.com

Source: https://www.wallstreetmojo.com/effective-interest-rate/

Share this post